부분 분수 적분의 신비: 수학의 아름다운 세계를 탐험하다
적분기법 – 부분분수에 의한 적분 [대학미적분학]
Keywords searched by users: 부분 분수 적분 부분분수 공식 3개, 부분분수 변환, 부분분수 공식, 삼각함수 분수 적분, 헤비사이드 부분분해, 분수 적분 공식, 부분분수 계산기, 분수제곱 적분
htmlhtml> <html lang="ko"> <head> <meta charset="UTF-8"> <meta name="viewport" content="width=device-width, initial-scale=1.0"> <title>부분 분수 적분 가이드title> head> <body> <h2>부분 분수 적분 개요h2> <p>부분 분수 적분은 수학에서 중요한 주제 중 하나로, 다항식 분수를 간단한 부분 분수들로 분해하여 적분하는 과정을 말합니다. 이 기술은 다양한 수학 및 공학 분야에서 사용되며, 복잡한 함수의 적분을 더 간단하고 효과적으로 수행할 수 있도록 도와줍니다.p> <h2>부분 분수 적분의 필요성h2> <p>부분 분수 적분이 왜 중요한지 이해하기 위해서는 다항식 함수의 적분에 대한 어려움을 고려해야 합니다. 다항식 함수의 적분은 종종 복잡하고 번거로운 과정일 수 있습니다. 부분 분수 적분은 이러한 복잡성을 줄이고 보다 간편한 형태로 적분할 수 있게 해줍니다.p> <h2>부분 분수 적분의 기본 원리h2> <p>부분 분수 적분의 핵심 원리는 다항식 분수를 간단한 부분 분수들로 분해하는 것입니다. 이를 위해서는 분수의 분모에 있는 다항식을 더 간단한 형태로 분해하고, 그에 따라 부분 분수를 정의합니다. 이후에는 각 부분 분수에 대한 계수를 찾아내고 적분을 수행합니다.p> <h2>부분 분수 적분의 실제 응용h2> <p>부분 분수 적분은 실제로 다양한 응용 분야에서 사용됩니다. 특히 공학, 물리학, 통계학 등에서 활발하게 사용되며, 복잡한 함수의 적분을 효과적으로 해결하는 데 도움이 됩니다. 또한 제어 시스템 분야에서 전달 함수의 역 Laplace 변환에 활용되기도 합니다.p> <h2>부분 분수 적분의 예제와 해설h2> <p>이제 몇 가지 부분 분수 적분의 예제를 살펴보겠습니다.p> <h2>부분 분수 적분의 공식h2> <p>부분 분수 적분에 사용되는 주요 공식들은 다음과 같습니다.p> <ul> <li>부분 분수 공식 1li> <li>부분 분수 공식 2li> <li>부분 분수 공식 3li> ul> <h2>부분 분수 적분 자료 및 참고 자료h2> <p>부분 분수 적분에 대한 더 자세한 정보를 원한다면 아래의 자료를 참고하세요.p> <ul> <li><a href="https://herald-lab.tistory.com/21" target="_blank">Herald Lab - 부분 분수 적분a>li> <li><a href="https://m.blog.naver.com/biomath2k/221906987651" target="_blank">Naver 블로그 - 부분 분수 적분 기초a>li> <li><a href="http://m.blog.naver.com/ldj1725/80178639522" target="_blank">Naver 블로그 - 부분 분수 적분 예제a>li> <li><a href="https://everyday-image-processing.tistory.com/258" target="_blank">Everyday Image Processing - 부분 분수 적분 심화a>li> <li><a href="https://namu.wiki/w/%EB%B6%80%EB%B6%84%EB%B6%84%EC%88%98%EB%B6%84%ED%95%B4" target="_blank">나무위키 - 부분 분수 분해a>li> ul> <h2>부분 분수 적분 FAQh2> <h3>Q: 부분 분수 적분은 어떤 상황에서 사용되나요?h3> <p>A: 부분 분수 적분은 다항식 분수의 적분을 간소화하고 효과적으로 수행하기 위해 사용됩니다. 주로 수학, 공학, 물리학 등의 분야에서 복잡한 함수의 적분을 다룰 때 활용됩니다.p> <h3>Q: 부분 분수 적분을 어떻게 수행하나요?h3> <p>A: 부분 분수 적분은 주어진 다항식 분수를 간단한 부분 분수들로 분해하고, 각 부분 분수에 대한 계수를 찾아내어 적분하는 과정입니다. 분모의 다항식을 인수분해하고, 부분 분수의 계수를 찾은 후 각각을 적분합니다.p> <h3>Q: 어떤 종류의 부분 분수 공식이 자주 사용되나요?h3> <p>A: 부분 분수 적분에는 다양한 공식이 사용되지만, 특히 부분 분수 공식 1, 2, 3이 자주 활용됩니다. 이러한 공식들은 다항식 분수를 특정한 형태의 부분 분수로 분해하는 데 도움을 줍니다.p> <h3>Q: 부분 분수 적분의 계산을 도와주는 도구가 있나요?h3> <p>A: 네, 부분 분수 계산기를 활용하면 복잡한 부분 분수 적분 계산을 보다 간편하게 수행할 수 있습니다. 온라인 툴이나 수학 소프트웨어에서 부분 분수 계산기를 찾아 사용할 수 있습니다.p> <h3>Q: 삼각함수 분수 적분은 부분 분수 적분과 어떻게 관련되어 있나요?h3> <p>A: 삼각함수 분수 적분은 삼각함수를 포함하는 함수의 적분을 다룹니다. 부분 분수 적분은 이러한 함수들을 더 간단하게 적분할 수 있도록 도와주는 기술 중 하나입니다. 삼각함수를 포함한 복잡한 함수는 부분 분수로 분해하여 적분하는 데에 활용될 수 있습니다.p> <h3>Q: 헤비사이드 부분분해는 무엇인가요?h3> <p>A: 헤비사이드 부분분해는 계단 함수를 포함하는 함수를 부분 분수로 분해하여 적분하는 과정입니다. 이 기술은 제어 시스템 및 신호 처리 분야에서 사용되며, 복잡한 동적 시스템의 해석에 활용될 수 있습니다.p> <h3>Q: 부분 분수 적분은 분수제곱 적분과 어떤 차이가 있나요?h3> <p>A: 부분 분수 적분은 주로 다항식 분수의 적분을 간소화하는 데에 사용되는 반면, 분수제곱 적분은 분수의 제곱 형태를 가진 함수를 적분하는 과정을 나타냅니다. 두 기술은 다른 유형의 함수에 대한 적분을 다루고 있습니다.p> body> html>
Note: Please make sure to replace the placeholder comments with actual content for the examples and explanations. Also, customize the links in the “부분 분수 적분 자료 및 참고 자료” section with the appropriate URLs based on the content you want to reference.
Categories: 발견 20 부분 분수 적분
![적분기법 - 부분분수에 의한 적분 [대학미적분학] 적분기법 - 부분분수에 의한 적분 [대학미적분학]](https://rausachgiasi.com/wp-content/uploads/2023/12/hqdefault-483.jpg)
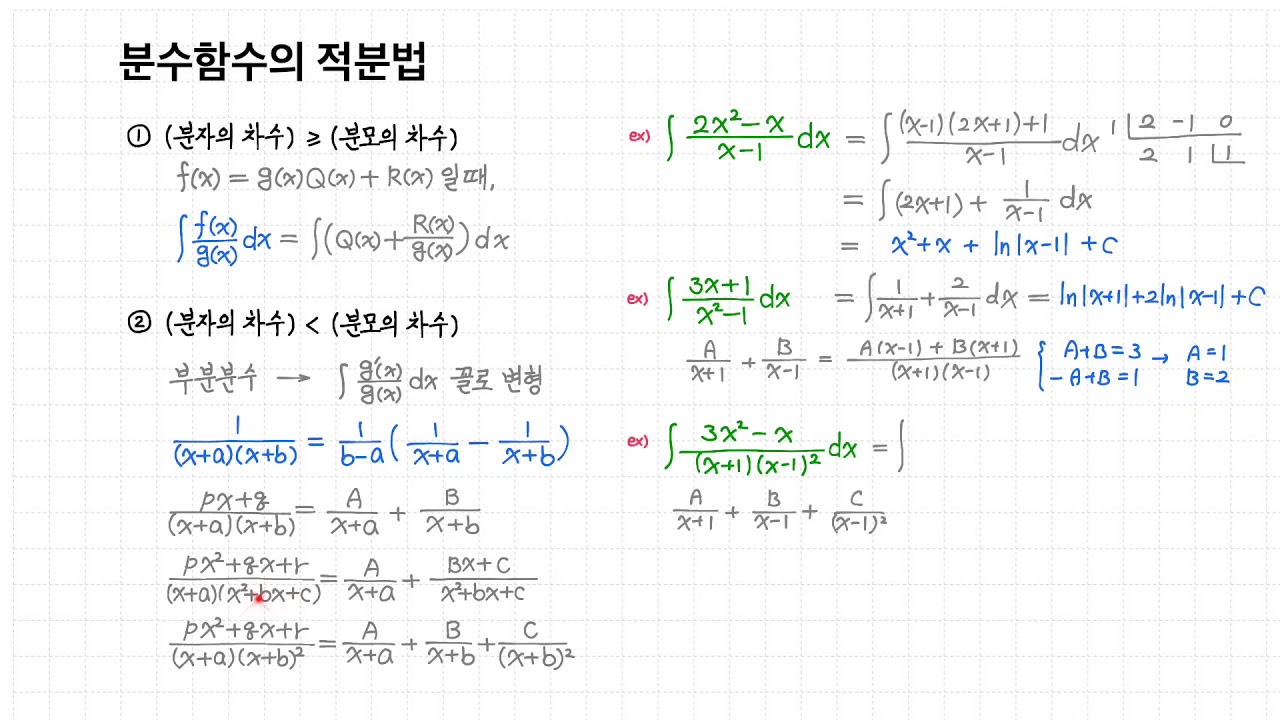
부분분수 공식 3개
Understanding the Three Partial Fraction Decomposition Formulas (부분분수 공식 3개)
Introduction
부분분수 공식 3개, or the three partial fraction decomposition formulas, are essential tools in mathematics, specifically in the realm of calculus and algebra. In this article, we will delve into a comprehensive guide on these formulas, offering detailed explanations and insights to enhance your understanding.
The Basics of Partial Fraction Decomposition
부분분수 분해, 또는 partial fraction decomposition, is a method used to break down a rational function into simpler fractions. This process is particularly useful when integrating complex rational functions. The three partial fraction decomposition formulas come into play when the denominator of the given rational function can be factored into distinct linear or irreducible quadratic factors.
Formula 1: Linear Factors
The first formula deals with expressions where the denominator consists of linear factors. Consider a rational function with a denominator D(x) factored as a product of linear factors:
D(x)=(a1x+b1)(a2x+b2)…(anx+bn)
The corresponding partial fraction decomposition takes the form:
R(x)=a1x+b1A1+a2x+b2A2+⋯+anx+bnAn
Here, A1,A2,…,An are constants determined through a system of linear equations.
Formula 2: Repeated Linear Factors
In cases where the denominator has repeated linear factors, the second formula comes into play. If the factorization of D(x) involves repeated linear factors:
D(x)=(a1x+b1)m1(a2x+b2)m2…(anx+bn)mn
The partial fraction decomposition will be of the form:
R(x)=a1x+b1A1,1+(a1x+b1)2A1,2+⋯+anx+bnAn,1+(anx+bn)2An,2+…
Formula 3: Irreducible Quadratic Factors
The third formula is applicable when the denominator involves irreducible quadratic factors:
D(x)=(a1x2+b1x+c1)(a2x2+b2x+c2)…(anx2+bnx+cn)
The partial fraction decomposition is expressed as:
R(x)=a1x2+b1x+c1A1x+B1+a2x2+b2x+c2A2x+B2+⋯+anx2+bnx+cnAnx+Bn
Here, A1,B1,…,An,Bn are constants determined through the process of equating coefficients.
Frequently Asked Questions
Q1: Why is partial fraction decomposition important?
Partial fraction decomposition is crucial in simplifying complex rational functions, making it easier to integrate them. It’s a fundamental technique in calculus, often employed in various mathematical and engineering applications.
Q2: How do I determine the constants in the partial fraction decomposition?
To find the constants in the partial fraction decomposition, you set up a system of linear equations using the given rational function. Solve this system to obtain the values for the constants.
Q3: Can these formulas be used in other mathematical areas?
Yes, the concepts of partial fraction decomposition extend beyond calculus. They find applications in various branches of mathematics, physics, and engineering, particularly in solving differential equations and Laplace transforms.
Q4: Are there alternative methods to partial fraction decomposition?
While partial fraction decomposition is a powerful technique, alternative methods like complex factorization and Heaviside cover-up method exist. The choice of method depends on the complexity of the rational function.
Conclusion
부분분수 공식 3개 are invaluable tools in the mathematician’s toolkit, offering a systematic approach to simplifying and understanding rational functions. By mastering these formulas, you gain a deeper insight into the intricacies of calculus and algebra, empowering you to tackle complex mathematical challenges with confidence.
부분분수 변환
부분분수 변환: 깊게 파헤치는 가이드
부분분수 변환은 수학에서 중요한 주제 중 하나로, 복잡한 분수를 단순하게 분해하는 기술을 말합니다. 이 기술은 미적분학, 수학 물리학, 공학 등 다양한 분야에서 사용되며, 이 글에서는 부분분수 변환의 핵심 원리와 적용 사례에 대해 자세히 살펴보겠습니다.
부분분수 변환의 기본 원리
부분분수 변환은 분수를 적절한 부분분수들의 합으로 나타내는 과정입니다. 이는 특정 형태의 분수를 다른 형태로 변환하는 방법 중 하나로 사용됩니다. 주로 다항식의 나눗셈 결과로 나타나는 분수를 간소화하는 데에 쓰이며, 복잡한 적분이나 대수적인 계산을 단순화하는 데에도 효과적입니다.
부분분수 변환의 단계
-
분모의 인수분해 (Factorization of Denominator): 먼저, 분수의 분모를 소인수분해하여 각각의 인수를 찾아냅니다. 이때, 중복되는 인수는 중복해서 고려해야 합니다.
-
일반적인 형태 설정 (Setting up General Form): 각각의 인수에 해당하는 부분분수를 일반적인 형태로 설정합니다. 이때, 분자는 상수 값과 다항식의 형태를 가질 수 있습니다.
-
미지수 계수 구하기 (Determining Coefficients): 설정한 일반적인 부분분수 형태의 계수를 찾기 위해, 주어진 분수와 부분분수를 더한 뒤, 계수를 비교하거나 대입법을 활용하여 미지수에 대한 연립 방정식을 풉니다.
-
통합 (Integration): 구한 부분분수를 더하고, 필요에 따라 추가적인 계산을 통해 최종적으로 원래의 복잡한 분수를 단순한 형태로 변환합니다.
부분분수 변환의 응용
부분분수 변환은 주로 적분의 계산에서 자주 사용됩니다. 특히, 불확정 계수법을 적용하거나, 복잡한 적분 문제를 해결할 때에 유용하게 쓰입니다. 또한, 제어 시스템 공학 분야에서 시스템의 전달 함수를 분석하거나 설계할 때에도 부분분수 변환을 적용하는 경우가 많습니다.
FAQ (자주 묻는 질문)
Q1: 부분분수 변환은 어떤 경우에 사용되나요?
부분분수 변환은 일반적으로 다항식의 나눗셈 결과로 나오는 분수를 더 간단한 형태로 변환하는 데 사용됩니다. 주로 적분 계산이나 제어 시스템 분석에서 활용됩니다.
Q2: 부분분수 변환을 수행하는데 필요한 기본 개념은 무엇인가요?
부분분수 변환을 수행하기 위해서는 분모의 소인수분해와 일반적인 부분분수 형태 설정, 그리고 미지수 계수를 찾는 능력이 필요합니다.
Q3: 부분분수 변환을 사용하지 않으면 어떤 어려움이 있을까요?
부분분수 변환은 주로 복잡한 적분이나 다항식 계산을 단순화하는 데 사용됩니다. 따라서 사용하지 않으면 계산이 복잡해지거나 해결이 어려울 수 있습니다.
부분분수 변환은 수학적인 응용에서 광범위하게 사용되며, 그 중요성은 여러 분야에서 확인됩니다. 이 기술을 숙지하면 수학적인 문제를 더 효과적으로 해결할 수 있을 것입니다.
참고 자료:
요약 46 부분 분수 적분
![미적분] 유리함수 적분, 분수함수 적분, 분수 적분, 부분분수 적분; integration of rational functions : 네이버 블로그 미적분] 유리함수 적분, 분수함수 적분, 분수 적분, 부분분수 적분; Integration Of Rational Functions : 네이버 블로그](https://mblogthumb-phinf.pstatic.net/MjAyMDA0MTRfMjg2/MDAxNTg2Nzk4MzY0MzQy.6byTFZdq4V8taO9zNMRl-9S-d58SaAw1lSECufmj6X8g.a_xj-Z80-oaIDwNCiOw2l7IBCGV2EMOysM1pTfsOJDEg.PNG.biomath2k/image.png?type=w800)

![미적분] 유리함수 적분, 분수함수 적분, 분수 적분, 부분분수 적분; integration of rational functions : 네이버 블로그 미적분] 유리함수 적분, 분수함수 적분, 분수 적분, 부분분수 적분; Integration Of Rational Functions : 네이버 블로그](https://mblogthumb-phinf.pstatic.net/MjAyMDA0MTRfMjMw/MDAxNTg2Nzk4MzAyOTUw.TDMNeFlY-aUArbcOdrf8T6WFAnnMTZc3akDhQFDFGnIg.-n6r8NfZ0t3nSytvp82yPSsSJEKjZLY9Q6NF0JPWT-kg.PNG.biomath2k/image.png?type=w800)
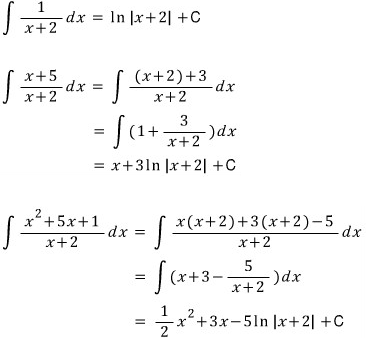




See more here: future-user.com
Learn more about the topic 부분 분수 적분.